The recent news of the great Indian batsman Sachin Tendulkar surpassing West Indian Brian Lara's record number of test runs has given maths-loving cricket geeks another opportunity to pull out their calculators and Excel spreadsheets. I'm openly one of these nuts and did just that.
At the time of writing, Tendulkar had scored 12,027 runs across 247 innings, to overtake Lara's 11,953 from 232 innings. After a little investigation, I found that despite his outstanding average of over 54 runs per innings, Tendulkar's most common score in test cricket is ... zero!
This was quite a shock — the most prolific run-scorer in test cricket has been out for nought (a duck in cricket parlance) 14 times, well ahead of his second most common score — which incidentally is the next lowest you can get: one!
This is completely counter-intuitive, so I took this investigation further. Australian cricketer Sir Donald Bradman is universally regarded as the best batsman ever to have played the game. His average, an astounding 99.94, is so far above every other batsman in the history of the game that he is often acclaimed as not only the best cricketer ever, but the best player ever of any sport. His average is so iconic in Australia that the postcode of the ABC (the Australian version of the BBC) is 9994 in every capital city. If it wasn't for the fact that much more test cricket is played nowadays than in the early 1900s, and for World War II interrupting his career for six years, Bradman would have scored many more than the 6996 runs he did score.
So, guess what Bradman's most common score was?
That's right, zero!
Indeed, looking at every innings by the most prolific batsmen in test history from Tendulkar at number 1 to Bradman at number 34, the most common score is zero — and by quite a long way too. The following figures show the distribution of scores from these top batsmen — on the horizontal axis you see the number of runs and the vertical axis measures the frequency of dismissals at a particular number of runs. The first chart shows every score between 0 and 100, and the second uses five-run wide bins to show scores up to 250. The data only include scores where the batsman was dismissed and so does not include not-out scores.

Scores plotted against dismissal frequency.
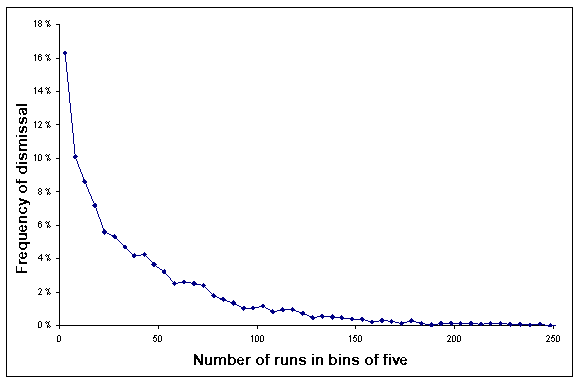
Scores in bins of five plotted against dismissal frequency.
Model cricket
A closer look at these distributions shows that they very closely fit what is known as an exponential distribution. An exponential distribution has the form
![\[ y=\lambda e^{-\lambda x}. \]](http://plus.maths.org/MI/67cae538615cf3236aecc880addb85a9/images/img-0001.png) |
In this case y is the probability of being dismissed at score x with λ being constant.
A common trick when looking at distributions involving exponentials is to take logarithms of both sides to get
![\[ ln(y) = ln(\lambda ) - \lambda x. \]](http://plus.maths.org/MI/67cae538615cf3236aecc880addb85a9/images/img-0005.png) |
The graph of this function, plotting ln(y) against x, is now a straight line with slope -λ. If the statistical data fits the exponential distribution, then the plot of the logarithm of the frequency of dismissals against the score at which dismissal happened should look roughly like a straight line.
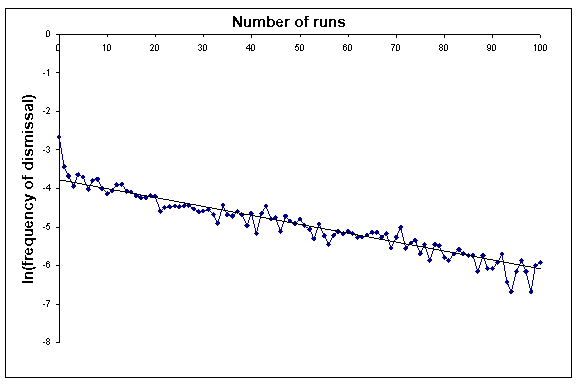
A straight line fitted to the data. The blue dots represent observed data and the black line represents the model.

A straight line fitted to the data from the second chart above. The blue dots represent observed data and the black line represents the model.
The mean of an exponential distribution, a sort of average, is 1/λ. In our case this gives a mean of around 43 - the same as we observe in the raw data. One can make the interesting observation that there is no such thing as the "nervous nineties": players do not "choke" and get out in the 90s, nervous before scoring a glorious test century, any more than they get out at any other score. Indeed, you could argue the opposite given the probability troughs at 94, 98 and in the 190s. You can also see that the probability of being dismissed for a duck is higher than you might expect for an exponential distribution.
So what?
Now, so far you might be thinking that all of this is only of passing statistical interest. So what if cricket scores follow an exponential distribution? Well, I'm glad you asked!
Let’s turn for a second to a different distribution, the geometric distribution. You will be familiar with this distribution from a simple 50/50 coin toss. The geometric distribution describes the number of coin tosses you need before a head (or tail) first turns up. The probability of your first head turning up on your kth toss is described as
![\[ Prob(first\; \; head \; \; on \; \; kth \; \; toss) = (1-p)^{k-1}p, \]](http://plus.maths.org/MI/622049c239be07d7fad2bbba4550a1f7/images/img-0002.png) |
where p is the probability of a head turning up on each toss, that is, 0.5. The distribution is memory-less, which is one of its key descriptors. No matter what has gone before, even if you have fluked 100 tails in a row, the probability of a head turning up on the 101st throw is still p.
The geometric distribution only works for integer values of k, that is, you can only throw a coin 2, 3, 100 etc times and not 2.5 times. The exponential distribution is the continuous equivalent of this distribution, extending it to work for all numbers, not just integers. Given that cricket batting scores seem to fit a exponential distribution, this means that we can picture cricket batting scores on a geometric distribution with the probability of you being dismissed at score as
![\[ Prob(dismissed\; \; at\; \; score\; \; k) = (1-p)^ kp. \]](http://plus.maths.org/MI/622049c239be07d7fad2bbba4550a1f7/images/img-0005.png) |
Can you spot the profound result here?
Remembering that the geometric distribution is memory-less, you can interpret this as saying that no matter what score you are currently on, you have the same chance p of getting out on that score as you do on any other score! Like a coin toss, the probability of you being dismissed on each score does not depend on what has gone before. A model which assumes that there is no memory is known as a constant hazard model.
This seems to go against every cricketing manual I have ever read. Accepted cricketing wisdom says that a batsman is more dangerous when (s)he "has the eye in" and has scored 10 or 20 runs. Our result seems to suggest that, apart from when a batsman is on 0, you have just as much chance of dismissing him or her on the current score as on any other score.
The next question to ask is, what is the probability of dismissing a batsman on the current score (that is, what is p in the above equation)? The mean of a geometric distribution is
![\[ mean = \frac{1-p}{p}. \]](http://plus.maths.org/MI/16b412cd9e368be9c558fc2e09e52f3f/images/img-0002.png) |
Knowing that the mean of the exponential distribution is 1/λ, and transferring this to the geometric distribution, we get
![\[ p = \frac{\lambda }{\lambda + 1}. \]](http://plus.maths.org/MI/16b412cd9e368be9c558fc2e09e52f3f/images/img-0004.png) |
For λ = 0.023 this gives p = 0.022. Therefore, if you were to turn the television on now and find the cricket coverage, the chance that the batsman you are watching gets out on the current score is 2.2%.
Scores near zero
The biggest deviation from the geometric distribution is for scores near zero. According to our data, the chance of being dismissed for a duck is 6.9% — around 3 times more than expected for a geometric (or exponential) distribution. But by the time the batsman has scored two or three runs, the geometric distribution starts to fit well. There is a small peak at four runs, perhaps because you can relatively easily get to four before you become comfortable — it only takes one streaky shot to the boundary. Whilst you can get to three with one shot, you are more likely to have played a few shots and so may be comparatively more "set".
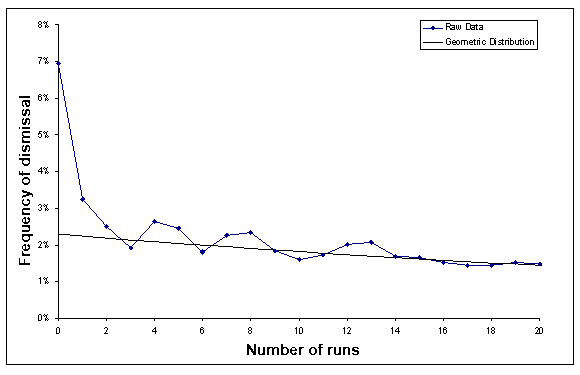
The data and the geometric distribution. The blue dots represent observed data and the black line represents the model.
By assuming a constant hazard model, Brewer determined the effective average of a batsman before they have scored — that is, assuming a constant hazard model with probability p of dismissal equal to that of their chance of being dismissed for a duck, Brewer determined the mean of this new distribution.
In our data from the best batsmen of all time, dismissal for a duck occurred with a 6.9% chance. The mean of a geometric distribution built around this probability is
![\[ \frac{1-0.069}{0.069} = 13.5. \]](http://plus.maths.org/MI/88a431ff6ada65ad2a1a1109694e7d0d/images/img-0001.png) |
This means that even though our batsmen have a mean of about 43, before they've scored they bat like cricketers with a mean of 13.5. Even the best batsmen bat like tail-enders before they get off the mark!
Conclusions
What should we take away from this analysis?
The conclusion seems to be that there is a very small window in the beginning of a batsman's innings in which there is a greater chance of dismissal than there ordinarily is. This makes sense — batsmen take some time to acclimatise to the game conditions. But this is a small window — once the batsman has scored about three runs, you have the same chance of dismissal whatever the current score. Interestingly, tiredness does not seem to play a part — the exponential distribution holds well out to 250 runs (quite a few hours of batting).
It should be remembered that this analysis was completed on the top 34 run scorers of all time (5953 innings) and so represents the best ever batsmen. Lesser batsmen are likely to get low scores, so perhaps this window is slightly wider for them. But if we turn to the greatest of the great, Bradman, the window is essentially one run. His effective average before he had scored was a very mediocre nine runs. After he had scored two runs, this effective average had risen to 69. You had to get Bradman out very early!
More information
- The data was retrieved from cricinfo during the second test between Australia and India on the 19th of October 2008;
- Not-out scores were removed from the analysis;
- The exponential distribution does break down a little for scores above 250 as there simply isn't enough data;
- Yes, Marc has scored a duck in his cricket career.
Further reading
- Read Brendon J. Brewer's paper Getting your eye in: A Bayesian analysis of early dismissals in cricket;
- Find out more on the maths/cricket blog Pappus' plane — cricket stats.
- Of course, see Plus!












Very interesting analysis (I've Dugg your submission). But I'm not sure if I follow your move from the exponential distribution to the geometric...
ReplyDeleteIf a batsman's scoring follows an exponential distribution, and his historical average per innings is 1/λ then:
P(he will score exactly x runs) = λexp(-λx)
If I understand correctly, your statement that "if you were to turn the television on now and find the cricket coverage, the chance that the batsman you are watching gets out on the current score is 2.2%" doesn't look right. The probability of getting out next ball isn't constant but depends on how many runs they've already made.
Hi Stanley - thanks for the comment, and the Digg!
ReplyDeleteYou are right, the batsman's scoring does follow an exponential distribution, but the statement re turning the tv is on is correct.
Imagine a coin tossed over and over again. The first time a head turns up follows an exponential distribution - like our batting dist - when the head turns up, you're out. There is a 50% chance it will turn up on the first toss. Given that 50% of the time a head has already turned up by the time we toss the coin a 2nd time, the probability that we actually toss the coin a 2nd time is 50% and the probability of that coin toss yielding a head is 50%, so overall, the probability of a head turning up on the 2nd toss is 50% times 50% - which is 25%.
The trick here is to remember that with a coin toss, at each toss, there is a 50% chance of a head turning up NO MATTER what has come before. Even if you have tossed the coin 100 times, the probability of a head on the 101st toss is 50%. It's the same the with batting, although instead of the probability involved being 50%, its around 2%.
Re moving from the exponential to the geometric dist, the exponential distribution is the continuous equivalent of geometric distribution, extending it to work for all numbers, not just integers. As you can only get out at integer values in cricket, the geometric distribution is the apt dist to use. For a decent data set like we have here, we can interchange the dists.
Thanks for reading!
marc
So Mike Hussey's recent run of low scores is not poor form, it's re-affirming his greatness..
ReplyDeleteI can think of a batsman that bucks the trend - although obviously I understand that he's an outlier. England's Alastair Cook (66 innings, 2 not outs)'s mode score is 60, which he's been out on five times, compared with just one duck. I guess a few other players (like AB DeVilliers, who's never had a duck) will also fall into a category like this. But yeah, they're definitely exceptions. Really good article :).
ReplyDeleteIt is a proficient article that you have shared here about cricket. I got some unique and valuable information from your article. Thankful to you for sharing this article here.Connect with Cricket grounds umpires and scorers
ReplyDeletestamped concrete
ReplyDeleteThe information you've provided is quite useful. It's incredibly instructional because it provides some of the most useful information. Thank you for sharing that. Buy Cricket Batting Pads Online
ReplyDeleteAmazing cricket game statistics. Keep this up. If you want to see newest trends in design for bathrooms check out our services.
ReplyDeleteIt is truly a well-researched content and excellent wording. I got so engaged in this material that I couldn’t wait to read. Read more info about cricket clothing online shopping. I am impressed with your work and skill. Thanks.
ReplyDeleteVery informative post. Nice blog content. Thanks for sharing. HVAC repair
ReplyDeleteIf it wasn't for the fact that much more test cricket is played nowadays than in the early 1900s, and for World War II interrupting his career for six years, Bradman would have scored many more than the 6996 runs he did score. about us
ReplyDeleteI'm so happy to found this site, I love science and I'm very curious about everything. https://www.inspectionmanaging.com/
ReplyDeleteIt's great to see this awesome information here. anchorirrigation.com/
ReplyDeleteImpressive analysis! It's such a great content. Thank you for sharing!
ReplyDeletewww.excavationdoneright.com/excavation-contractors-louisville-ky
Remembering that the geometric distribution is memory-less, you can interpret this as saying that no matter what score you are currently on. - basement crack repair
ReplyDeleteI definitely love discussions like this. Thank you!
ReplyDeletepool deck resurfacing Sarasota
The following figures show the distribution of scores from these top batsmen — on the horizontal axis you see the number of runs and the vertical axis measures the frequency of dismissals at a particular number of runs. home contractor
ReplyDeleteThank you for always sharing here an informative blog. info
ReplyDeleteThe data only include scores where the batsman was dismissed and so does not include not-out scores. | sunsetter dealer near me
ReplyDeleteInteresting article thank you for sharing check article here Securities Classaction Directory
ReplyDeleteThe probability of getting out next ball isn't constant but depends on how many runs they've already made.bathroom remodeling companies
ReplyDeleteAccepted cricketing wisdom says that a batsman is more dangerous when (s)he "has the eye in" and has scored 10 or 20 runs. Roofing company in el paso texas
ReplyDeleteI like the content of this blog, very informative one. https://www.americanheatingandairga.com/
ReplyDeleteTolerating for the time being that you're searching for an exhaustive manual for making a plans channel that devotees, you've come to the best regions. Deals pipes are one of the chief pieces of web progressing, and whenever done definitively, can make tremendous benefits for your business. In this article, we will examine the basics of game plans pipe movement, and give tips and deceives to making areas of strength for a that will change over programs into purchasers!
ReplyDeleteSales Funnel Development FL
Much appreciation to you for sharing these sythesis for a blog rules with making bloggers like us. Keep on sharing. Respects, https://messiahdfou373.substack.com/p/the-ultimate-cheat-sheet-on-viral?sd=pf
ReplyDeleteThis is much appreciated. I want to read more
ReplyDeleteThere is a small peak at four runs, perhaps because you can relatively easily get to four before you become comfortable — it only takes one streaky shot to the boundary. crawl spaces near me san francisco ca
ReplyDeleteIt's wonderful to see such amazing information presented here. I am overjoyed to have found this website. https://www.electricianvictoriabc.com/emergency-electrician
ReplyDelete
ReplyDeleteA common trick when looking at distributions involving exponentials is to take logarithms of both sides to get gmb listing
The following figures show the distribution of scores from these top batsmen — on the horizontal axis you see the number of runs and the vertical axis measures the frequency of dismissals at a particular number of runs. basement crack repair
ReplyDeleteRemembering that the geometric distribution is memory-less, you can interpret this as saying that no matter what score you are currently on. exterior painting services
ReplyDeleteNice! You can also see that the probability of being dismissed for a duck is higher than you might expect for an exponential distribution.
ReplyDeletePretty great post. I just stumbled upon your blog and wanted to say that I’ve truly loved browsing your blog posts.
ReplyDeletemoodle nottingham
moodle maisonneuve
Fantastic blog i have never ever read this type of amazing information. travis bickle jacket
ReplyDeleteI never thought this much math could be applied into Cricket of all things, it really goes to show how diehard some fans can be because I'm sure the common fan wouldn't go that far over some calculations related to the game. Thanks a lot for sharing this! please be sure to check out the best appliance repair company in Lubbock, TX.
ReplyDeleteThanks for this great information you shared. northalpestcontrol.com/
ReplyDeleteSuch an awesome post. Thanks for sharing! more
ReplyDeleteGlad to visit this site. Great work! debonnaireconstruction.com/
ReplyDelete
ReplyDeleteFor λ = 0.023 this gives p = 0.022. Therefore, if you were to turn the television on now and find the cricket coverage, the chance that the batsman you are watching gets out on the current score is 2.2%.
Kurt | drywall contractor
Thank you for sharing this very informative blog here. resolutions-av.com/
ReplyDeleteI'm Srilankan and I was named after this legend
ReplyDeletehttps://www.nswtreeloppers.com.au/
Hey! this is so informative. Thanks Awesome
ReplyDeleteThere is a small peak at four runs, perhaps because https://www.drywalldc can relatively easily get to four before you become comfortable.
ReplyDeleteYey! this is so informative. Thanks www.watersoftenergurus.com
ReplyDeleteSports fans really look into the stats! call us
ReplyDeleteI don't know why I took this very seriously. hahaha! I had so much fun reading it!
ReplyDeletenorelco beard trimmer
This is very informative. And to be honest, this is so fun to read about. Many thanks.
ReplyDeleteTampa best SEO company
This is great info for a beginner like me. southamptonfencingpros.com
ReplyDeleteSo far you have a good content! Thank you 3cre-commercial-real-estate.business.site/
ReplyDeleteWhat a fun article to read! contact us
ReplyDeleteGlad I was able to check this article! Science contents really interest me. contact us
ReplyDeleteAt this point why not just admit this is an excuse to avoid the wives? eastbournetreesurgeons.co.uk
ReplyDeleteWow! Thank you from dime method. I love your writing voice because it's engaging and approachable.
ReplyDeletePop culture and Science are two of my favorite things! call us
ReplyDeleteBy assuming a constant hazard model, Brewer and Pasadena Concrete Company determined the effective average of a batsman before they have scored.
ReplyDeleteYou are an awesome writer. I am grateful for this content. If you are looking for house painter near me in West Ashley, SC.
ReplyDeleteso far this is so incredible. Love the whole concept! https://atlanticsigncompany.com/business-signs-cincinnati-ohio/
ReplyDeleteJust read the whole article and found it really interesting! www.shopedc.com
ReplyDeleteThis finding challenges the assumption that the highest-scoring players would also have a correspondingly higher frequency of scoring runs. The charts provided in the investigation display the distribution of scores for the top batsmen, showing the frequency of dismissals at different run levels. superiordraincleaning.com/
ReplyDeleteCricket statistics, however, should be viewed as a complement to the overall cricketing experience, as they capture only a part of the game's essence. The thrill of watching a live match, the ebb and flow of emotions, and the camaraderie among fans are equally important aspects that make cricket a beloved sport.
ReplyDeleteThis is so informative! I love it. Thanks Check it out
ReplyDeleteBased on the search results, it seems that there is no specific term or topic called "cycling and kite core". However, there are some relevant results related to Core kites store and cycling. There are results about a kite fitness gym, kite skiing or snow-cycling, and kiteboarding as a watersport combined with cycling as a way of transportation. Is there a specific question or topic you want me to focus on related to kites and cycling? I will do my best to help if you can provide more specific information.
ReplyDeleteEven though players like Sachin Tendulkar and Sir Donald Bradman are renowned for their remarkable achievements and high averages, they have also experienced their fair share of failures in the form of ducks. Cricket is a sport that demands consistent focus and skill, and even the best players can have moments of vulnerability. superiordraincleaning.com/
ReplyDeleteI’m so excited to get started
ReplyDeleteCricket fans are a passionate bunch, and they love to talk about the game. One of the things that fans love to talk about is statistics. Cricket is a game of numbers, and there are stats for everything from batting averages to bowling averages to fielding percentages.
ReplyDeleteThank you for this site. I love your writing voice because it's engaging and approachable. All the best from our vinyl fence installation company Sioux City
ReplyDeleteYou have a very nice output! Thanks https://scottkeeverseo.com/
ReplyDeleteFocusing on professional skills enables people to get better at what they do. Cheers from wood fence installation in Texas.
ReplyDeleteWow, that was a comprehensive analysis of cricket batting scores and their distribution. It's fascinating to see how statistical models, such as the exponential and geometric distributions, can be used to understand and interpret the patterns in cricket scores. www.excavatingcincinnati.com
ReplyDeleteI also agree with your statement that the most passionate followers can recite each scorecard from this year's Wisden. Wisden is the bible of cricket, and it's a must-read for any serious cricket fan. The scorecards in Wisden are a great way to track the progress of a match, and they can also be a great way to learn about the history of cricket.
ReplyDeleteCheers from our vinyl pools company at Amarillo, Texas.
ReplyDeleteI love the context! Thanks https://coutureusa.com/collections/chanel
ReplyDeleteYey! this is so informative. Thanks click
ReplyDeleteI'm so happy with the content! Thanks https://www.unmannedsas.com/
ReplyDeleteHow can i connect Science in the Bible? I'm very curious about this two.
ReplyDeleteAbsolutely fascinating analysis! I'm amazed at how even the titans like Tendulkar and Bradman aren't immune to the "duck curse." Your use of statistical models to break down the probability of a batsman getting dismissed at various scores is enlightening. It challenges conventional wisdom about batsmen being "settled" after scoring a few runs and offers a fresh perspective on the game. It’s compelling to think that even the greats bat like tail-enders until they're off the mark! stenciled concrete mornington
ReplyDeleteWow, that's some deep dive into cricket stats! It's fascinating that even the greats like Tendulkar and Bradman are most commonly out for a duck. Makes the game feel a bit more unpredictable and exciting! Click here
ReplyDeleteFascinating read! Never thought that batting scores would fit an exponential distribution so well. Makes me rethink the whole "settled batsman" concept. Great analysis! gutter repair near me
ReplyDeleteFascinating analysis on how cricket scores follow an exponential distribution! The concept that batsmen have the same chance of dismissal regardless of their current score really goes against conventional wisdom. It's especially intriguing to learn that even legends like Tendulkar and Bradman had zero as their most common score. Thanks for shedding new light on the "curse of the duck" and the nuances of cricket statistics! concrete driveway near me
ReplyDeleteFascinating read! Surprising to see how stats challenge common cricket wisdom about when a batsman is most likely to get out. gutter repair northern beaches
ReplyDeleteYou have a nice output! Thanks unmannedsas.com/
ReplyDeleteKudos from our medicaresalesagent.com
ReplyDeleteThis is completely counter-intuitive, so I took this investigation further and .remove wall texture. Australian cricketer Sir Donald Bradman is universally regarded as the best batsman ever to have played the game
ReplyDeleteThank you for creating such incredible content. click
ReplyDeleteIt's fascinating to see how cricket's greatest batsmen, including Sachin Tendulkar and Sir Donald Bradman, have zero as their most common score. The statistical quirks of the sport never cease to amaze! business brokerage hurricane ut
ReplyDeleteIn cricket we have a lot of memories related to the curse of a duck and so many legendary great players faced it. You can visit us to find more amazing services that helps the people to find the perfect solutions.
ReplyDeleteWow, this is so good! Thank you Poseidon Fishing Charters
ReplyDeleteWow, it's crazy to think that even cricket legends like Tendulkar and Bradman had their fair share of ducks! pest control cottonwood heights ut
ReplyDeleteThere is a small peak at four runs, possibly because reaching four is comparatively easy for https://www.drywallmarietta before becoming comfortable - one streaky shot to the boundary can do the trick.
ReplyDeleteI adore this post. Cheers from our best medicare IMO
ReplyDeleteAs someone who's watched countless cricket matches, I've always heard commentators talk about the "nervous nineties" and how batsmen are more likely to get out just before reaching a century. It's intriguing to see this analysis challenging that notion, especially for the top-tier batsmen. The idea that even the best batsmen essentially "bat like tail-enders" before getting off the mark is quite a revelation and certainly adds a new dimension to understanding the game.
ReplyDeleteI adore this post and site is amazing. You are making a difference. Kindest regards from our Roof Contractor in Wentzville.
ReplyDeleteThe revelation that both Tendulkar and Bradman's most common score is zero is truly surprising, challenging conventional cricket wisdom. The analysis also sheds light on the brief vulnerability at the start of a batsman's innings, emphasizing the importance of acclimatization to game conditions.
ReplyDeleteI appreciate your practical tips. I'm going to start using them right away. | https://enjoymexico.net/
ReplyDeleteYou absolutely nailed it. It was so satisfying. Cheers from our Cape Girardeau Remodeling Contractor Cape Girardeau at https://www.capegirardeauremodeling.com/
ReplyDeleteI completely agree with this article! Resin driveways and pavement provide a number of advantages over traditional alternatives. EdinburghPaving
ReplyDeleteI think I also experience the same when I'm on my highscool auto repair gilbert az
ReplyDeleteVaping Fryd wholesale devices work by heating the e-liquid, turning it into vapor. Users inhale this vapor, which often contains nicotine, flavorings, and other chemicals.
ReplyDeleteThis is amazing. Kudos and learn more from our buffalohealthadvisors.com
ReplyDeleteWhether you're a skeptic seeking to unravel the truth behind the legend or a believer intrigued by the supernatural, join us as we embark on a chilling journey to decipher The Curse of the Duck—an enigma that continues to captivate the curious minds and spark whispers in the dark.
ReplyDeletebankruptcy lawyers near me now
I found out my cousin plays this game.
ReplyDeletehttps://www.compare1.com.au/
Haha, that's a fascinating cricket stat! Who would've thought that the most common score for the greatest batsmen is zero? Cricket never ceases to amaze with its quirks. ac installation phoenix
ReplyDeleteIt's fascinating how cricket stats can reveal surprising insights! 🏏 Who would've thought that even legends like Sachin Tendulkar and Don Bradman had zero as their most common score? Cricket truly is full of intriguing facts and figures. stump grinding englewood co
ReplyDeleteCricket statistics can often reveal fascinating quirks in the game. It's intriguing to learn that some of the greatest batsmen in the sport, including Sachin Tendulkar and Sir Donald Bradman, have had zero (duck) as their most common score. The unpredictability of cricket is part of what makes it so captivating for fans and enthusiasts alike. 🏏📊 #CricketStats #Duck #SachinTendulkar #SirDonBradman bathroom remodeling cleveland tx
ReplyDeleteFascinating insights into the world of cricket stats! It's astonishing to see how even legendary batsmen like Sachin Tendulkar and Sir Donald Bradman had zero as their most common score. Cricket's unpredictability always keeps us intrigued, and this analysis adds another layer to the game's rich history. 🏏📈 #CricketStats #ZeroScore #SachinTendulkar #SirDonBradman ginseng benefits
ReplyDeleteCricket's allure lies in its intricate statistics, and this exploration of zero scores among cricket legends is both surprising and thought-provoking. It's a testament to the unpredictability of the game and the exceptional resilience of players like Tendulkar and Bradman. Cricket truly is a sport full of intriguing stories and records! 🏏📊 #CricketLegends #ZeroScores #CricketStatistics uti treatment at home
ReplyDeleteCricket statistics never cease to amaze! 🏏 It's fascinating to see that even legendary batsmen like Tendulkar and Bradman had zero as their most common score. Goes to show that cricket is full of surprises and quirks. Thanks for sharing this intriguing insight into the world of cricket stats! roof repair miami fl
ReplyDeleteThanks for every thing you did in our life I proudly say that I lived in his era.
ReplyDeletehttps://www.ausluxuryhire.com.au/
Science has a lengthy history of being taught and popularized. I believe a library or an online/Google search will yield a lot of leads.
ReplyDeleteBusiness Listings
Skateboarding Flow Bindings: where creativity meets concrete.
ReplyDeleteI read about the curse-of-duck in cricket and it is good to read about it here again. You are good to share the detail and we can find more ideas about this game of gentleman. While searching for the dam construction ideas I came here to see these amazing ideas you shared.
ReplyDeleteOnline vet services provide peace of mind knowing help is just a click away. services provide peace of mind knowing help is just a click away.
ReplyDeleteThis article humorously explores the "curse of the duck" phenomenon, offering a lighthearted take on a seemingly random yet intriguing topic. Clearwater Concrete
ReplyDeleteSuch an informative blog! http://rdmedicalproducts.com/transdermal-patch-manufacturer/
ReplyDeleteThis is a concept related to probability and statistics, exploring the idea in an engaging and informative manner. If you need Concrete Patio Augusta, call us today!
ReplyDeleteThis is awesome. Nice to know. All the best from our seniors funeral insurance
ReplyDeleteOne can make the interesting observation that there is no such thing as the "nervous nineties". drywall contractor players do not "choke" and get out in the 90s, nervous before scoring a glorious test century, any more than they get out at any other score.
ReplyDeleteNice and interesting topic. Check out more details - Concrete Driveway Augusta
ReplyDeleteGlad to check this out! enjoymexico.net
ReplyDeleteThanks for the great content you shared. residential construction
ReplyDeleteThe blog post on Mr. Science Show appears to discuss the "Curse of the Duck," likely exploring a humorous or peculiar topic related to ducks. Stamped Concrete Lawrenceville
ReplyDeleteThe analysis of cricket statistics reveals counter-intuitive trends such as the prevalence of zero as the most common score among the top batsmen in test history. This data underscores the unpredictable nature of cricket, where even the greatest players can frequently experience early dismissals. For cricket enthusiasts, these insights add another layer of depth to their appreciation of the game and the remarkable achievements of players like Sachin Tendulkar and Sir Donald Bradman. www.themyndclinic.com/
ReplyDeleteFascinating stat about Tendulkar! Even the greatest batsmen get dismissed for ducks sometimes. Makes you wonder - is consistency the key to high run scoring, or racking up big scores to balance out the occasional dismissal? Looking forward to finding out Bradman's most common score too! #CricketStats #TheDon #Sachin
ReplyDeleteConcrete Patio Goodyear
ReplyDeleteThis was a fascinating read about the "Curse of the Duck"! It's intriguing how certain sports superstitions can take on a life of their own. Have there been any recent instances where the curse seemed to make an impact?
Did you know that Sachin Tendulkar, one of cricket's greatest batsmen, is the only player to have scored 100 international centuries across Test and One Day International matches?
ReplyDeleteMany thanks for sharing this with everyone; you clearly know what you're talking about.
ReplyDeleteinsulationvancouver.ca
The blog post on Mr. Science Show discusses the "Curse of the Duck," exploring the origins and stories behind this intriguing topic.
ReplyDeleteLooking for Concrete Driveway Lawrenceville? Check it out!
Cricket fans love their stats because they provide a detailed and fascinating way to analyze player performance, track records, and uncover trends that enhance their appreciation of the game. If interested, please visit us at Tree Removal Kamloops
ReplyDeleteI'm so impressed with the content! Thank you
ReplyDeletemasonry contractorO
Interesting...thanks for the post. This blog has been saved! Chilliwack House Cleaning
ReplyDeleteAmazing! look how great this blog is.
ReplyDeleteclick
It's fascinating to see how even the greatest cricketers, like Tendulkar and Bradman, share the common experience of frequent ducks, illustrating that even top performers are susceptible to early failures. The analysis revealing that dismissal probabilities align with an exponential distribution is a compelling insight into the nature of batting and dismissal in cricket.
ReplyDeleteThe blog post discusses the "Curse of the Duck," a humorous or mysterious topic related to ducks. It explores the peculiarities or myths surrounding ducks, possibly blending science with a touch of whimsy. View more about Concrete Patio Port Saint Lucie.
ReplyDeleteYou have a good content! | silt fences
ReplyDeleteHappy to visit this one! Such an informative one. Visalia Fence Company Custom Fence
ReplyDeleteYou have a great blog! silt fences
ReplyDelete
ReplyDeleteHi there I am so thrilled I found your website, I really found you by mistake, while I was browsing on Yahoo for something else, Anyhow I am here now and would just like to say thanks a lot for a tremendous post and a all round exciting blog (I also love the theme/design), I don’t have time to go through it all at the minute but I have saved it and also added in your RSS feeds, so when I have time I will be back to read more, Please do keep up the awesome job. Visit our Commercial Cleaning Prince George for more services needed. Thanks
You really have a great blog! Memphis Home Painters
ReplyDeleteWow, this analysis really dives deep into cricket stats, and it’s wild to think that even legends like Tendulkar and Bradman have those zero scores lurking in their records. It kind of puts the pressure of the early innings into perspective, doesn’t it? If you’re curious to learn more about our services, feel free to check us out at Kamloops Painter.
ReplyDeleteYou have a nice output! | Alabama Artificial Grass Birmingham AL
ReplyDeleteYou have a good data! Thanks Hendersonville Concrete Company concrete contractor
ReplyDeleteWhat an informative blog! Thanks for this one. silt fences
ReplyDeleteYou have a good content! | scottkeeverseo.com/
ReplyDeleteThis is nice! Such an informative blog. Conway Concrete Company concrete patio
ReplyDeleteIt might dive into quirky facts, superstitions, or events related to ducks or use ducks as a metaphor for something unexpected. Find out more about the trusted Concrete Driveway Lawrenceville.
ReplyDeleteThank you so much for sharing this with us. concrete company
ReplyDeleteQuite excellent blog post. When i only happened at your weblog in addition to needed to say i always have really loved reading your website articles. Roofers Vancouver
ReplyDeleteYou have a good content! thanks for sharing contact us
ReplyDeleteThis is cool. If you also want cool and affordable lead generation ideas, visit us here at https://exclusiveleadstore.com/
ReplyDeleteKeep on sharing such an interesting blog! Savannah Concreters concrete patio
ReplyDeleteSuch an amazing blog. brick mason
ReplyDeleteKeep on sharing such an interesting blog! demolition contractor
ReplyDeleteThe fact that nobody from Bradman era has even close to half of Bradmans average in batting explains what Sir Bradman is all about. https://www.ausupersolutions.com.au/
ReplyDeleteThe fact that zero remains the most common score across prolific batsmen illustrates the relentless unpredictability and competitiveness of cricket. No matter how skilled or iconic, all players face a significant risk of early dismissal. This insight into batting scores showcases the game’s unique mixture of skill, strategy, and the inherent randomness that keeps fans — and statisticians — hooked. cincinnatiseo.org/
ReplyDeleteThe article shares amusing anecdotes where rubber ducks were suspected of bringing mishaps, adding a lighthearted twist to lab culture. Get in touch with Concrete Company Goodyear.
ReplyDeleteI never would've guessed that even the greats like Tendulkar and Bradman are so prone to getting out for a duck. It's wild that their most common score is zero, considering how much they've accomplished. I always assumed they'd be more consistent with runs, but I guess even the best have their off days.
ReplyDeleteAbsolutely! Cricket is a sport rich in statistics. It's fascinating how fans can immerse themselves in the numbers, analyzing every detail of a match. Whether it's batting averages, bowling figures, or historical records, cricket stats add depth and excitement to the game.
ReplyDeleteMicroCloud Blade Servers
Glad to find this blog! https://haroclean.com/
ReplyDeleteI went to Australia as an exchange student from the States and had no idea what Cricket was
ReplyDeletehttps://www.lux-creatives.com/
I’m usually to running a blog and i actually recognize your content. The article has really peaks my interest. I’m going to bookmark your web site and hold checking for brand spanking new information. Vancouver Car Detailing
ReplyDeleteKeep on sharing such an interesting blog! Big thanks from brick mailbox
ReplyDeleteYou have my gratitude for imparting such useful knowledge. Thanks for providing a trustworthy resource for door maintenance advice. My favorite part was the section on fixing common problems. ADA Handicap door chicago
ReplyDeleteWhen it comes to transportation, nothing beats the experience of riding in a limousine. Recently, I had the opportunity to ride one for a special event, and it was without a doubt the most incredible thing I have ever experienced. The voyage was made more delightful by the limo's comfortable interior as well as the ambient lights. Is there anything else that may have occurred during your excursion in the limousine? Ohare Limo Service
ReplyDeleteYou will be familiar with this distribution from a simple 50/50 coin toss. As a drywall consultant, this is pretty easy to understand.
ReplyDeleteReading by means of your enjoyable dispatch, desire further me to do so sometimes. plumberlangleybc.com
ReplyDeleteI've been finding this blog! Thanks for sharing this with us. Roofing contractor
ReplyDeleteLearned a lot from this blog! pool screening
ReplyDeleteAs the top concrete contractors in Savannah, GA, we are proud of our talented staff, who perform well on commercial and residential projects. All facets of concrete work are within our area of competence, including strong foundations, long-lasting concrete slabs, and striking stamped patios. We also offer trustworthy repair services to keep your concrete surfaces in top shape for many years. Concrete is a low-maintenance, long-lasting alternative for various uses, making it an excellent choice for companies and homes. Being one of the best concrete firms in Savannah, we are committed to using only the best materials and producing exceptional results on every project. For all your concrete needs, rely on us as your go-to cement contractor in Savannah, Georgia.
ReplyDeleteInteresting post. Thanks for sharing.
ReplyDeletehttps://healthvibed.com/spooky2-scalar-review/
This milestone reignited enthusiasm among cricket stat enthusiasts, prompting deeper dives into his career numbers. Surprisingly, despite his stellar average of over 54 runs per innings, Tendulkar’s most frequent test score is ... zero! He has been dismissed for a duck 14 times, which surpasses his second most common score, one.
ReplyDeleteThis revelation challenges expectations for a player widely regarded as one of the greatest batsmen. It underscores the quirks of statistical patterns, where frequent low scores coexist with an overall record of unmatched excellence. learn more
Bradman would have scored many more than the 6996 runs he did score in Jacksonville.
ReplyDeleteI've been finding this blog! Thanks for sharing this with us. anti aging treatments
ReplyDeleteYou have a great blog! Stamped concrete contractors
ReplyDeleteI like the helpful information you provide on your articles. www.electricianreddeer.com
ReplyDeleteThis is great! Happy to visit this kind of blog. commercial dumpsters
ReplyDeleteKeep on sharin this amazing blog! brick mason
ReplyDeleteThis is great! Glad to check this out. concrete sidewalk
ReplyDeleteYou have a great and interesting blog to visit! roof installation
ReplyDeleteYou can also see that the probability of being dismissed for a duck is higher than you might expect for an exponential distribution. See Baltimore Drywall Contractors for more information.
ReplyDeleteThis was a great article! Thank for taking time to share it. concrete contractors in Woodstock GA
ReplyDeleteVery interesting. I'm glad I stumbled on this. concrete driveway repair
ReplyDeleteI defiantly learned something
ReplyDeleteFascinating analysis! Even the greatest batsmen have their fair share of ducks—just shows how tough Test cricket is. The data breakdown really puts things in perspective. Thanks for sharing this insight!
ReplyDeleteThat’s an interesting read! The mix of science, superstition, and quirky history makes for a fascinating story. Did you find the "Curse of the Duck" theory convincing, or do you think it’s just a fun coincidence?
ReplyDeleteCheck out if the curse can trespass the Augusta Fencing Company.
It was illuminating to read this. It has given me great joy to discover your blog! Commercial Door Repair
ReplyDeleteKeep on sharing such an interesting blog! brick fireplace
ReplyDeleteSo glad I stumbled upon your blog. Every post brings something new and exciting! Abbotsford Carpet Cleaning
ReplyDeleteThe curse of the duck is a nice detail, and modern day cricket is not as easy as we think. Players need to work hard and practice more to be skilled enough to score a hundred. By using roofrestorationredlandbay.com.au I saw how the people are getting the right solutions to their problems.
ReplyDeleteThank you so much for sharing this informative blog with us. brick fireplace
ReplyDeleteKeep on sharing such an interesting blog! retaining wall
ReplyDeleteHe has been dismissed for a duck 14 times for basement insulation, which surpasses his second most common score, one.
ReplyDeleteVery interesting read — especially the bit about Bradman’s most frequent score being zero. I never would’ve guessed that! Stats like this really highlight how deceptive averages can be, and how important context is when analysing performance. Oprit installatie Zoersel
ReplyDeleteThank you so much for taking the time on sharing this with us. brick mason
ReplyDeleteYour writing style is so engaging. Loved every part of this! Commercial cleaning surrey
ReplyDeleteThis post about the "Curse of the Duck" is a fun and quirky dive into scientific skepticism, reminding us how superstition can sneak into even the most rational places. It’s a great reminder to keep a curious but critical mind. Check out also this trusted fence company
ReplyDeleteGod, thank you! In my opinion, the most crucial element influencing a student's ultimate grade in any given topic is their capacity to follow the course material. You might not have much time to study for the test if you disregard the course outline. Think of it as a timed study guide that indicates the precise amount of time required for each subject. Before the test, review the material once again. It typically outlines the primary objectives and regions to be assessed. Following the course plan and reviewing often will make exam preparation easy. Airport Service Limo
ReplyDeleteThank you so much for taking the time on sharing this with us. asphalt driveway
ReplyDeleteSuch a thoughtful perspective—definitely gave me something to think about. Lighting Kelowna
ReplyDeleteThank you for taking the time on sharing this with us. commercial door installation
ReplyDeleteOnce an electronic door lock stops working, how can I best troubleshoot it? On that matter, I might greatly benefit from your advice. handicap door openers
ReplyDelete